3.2 The Law of Mass Action

Notice in the previous section that the interaction between the lynx and the hare was of the form bHL - meaning you needed both positive values of H and L for the interaction to continue. This law states that the rate of a change is directly proportional to the product of the populations.

This assumption of the law of mass action is also commonly used in chemical reactions - especially in modeling enzyme dynamics. For example let’s say you have a substrate A that reactions with enzyme B to form a product S. Perhaps you might have seen this represented as a reaction equation:

\[\begin{equation} A+B \rightarrow S \end{equation}\]

How we would write the product of formation, or \(\displaystyle \frac{dS}{dt}\) is the following:

\[\begin{equation} \frac{dS}{dt}= kAB, \end{equation}\]

where \(k\) is the proportionality constant or the rate constant associated with the reaction. If we wanted to represent this as a schematic we would have the following diagram (Figure 3.4):

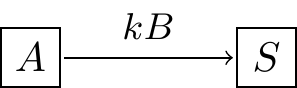
Figure 3.4: Schematic diagram of the law of mass action.

We could also consider if there was a constant decay of the substrate, which we might revise Figure 3.4 to Figure 3.5:

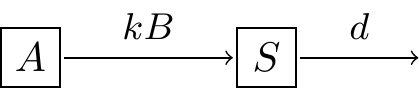
Figure 3.5: Revised schematic diagram of the law of mass action with decay.

For this case, the rate of change of \(S\) would then be:

\[\begin{equation} \frac{dS}{dt}= kAB - dS, \tag{3.4} \end{equation}\]

You may be wondering about rates for \(A\) and \(B\). When \(S\) is formed \(A\) and \(B\) are catalyzed, so the rate of formation for \(S\) (the positive term in Equation (3.4)) will be a loss for \(A\) and \(B\) (Equation (3.5)):

\[\begin{equation} \begin{split} \frac{dA}{dt} &= -kAB \\ \frac{dB}{dt} &= -kAB \end{split} \tag{3.5} \end{equation}\]

Both equations are similar in this case, not pending any additional inputs or outputs.